Welcome to my research portal – mathematical physics, machine learning (ML) & artificial intelligence (AI)

 
My scientific advances in mathematical physics lie at the intersection of  formal  aspects of stringy i.e. quantum gravity low energy physics and the phenomenology of vacuum structures in string theory and  cosmology. Utilizing techniques of applied mathematics such as algebraic and differential geometry.
The research heavily relies on  computational techniques which more recently led me to focus on the vibrant field of machine learning and artificial intelligence. 
My  recent  PostDoc research affiliation: Kavli IPMU  at the University of Tokyo, Japan 2015-Nov 2019

 

For my  advances (Jan 2020)  in the field of machine learning/AI, see here .

 
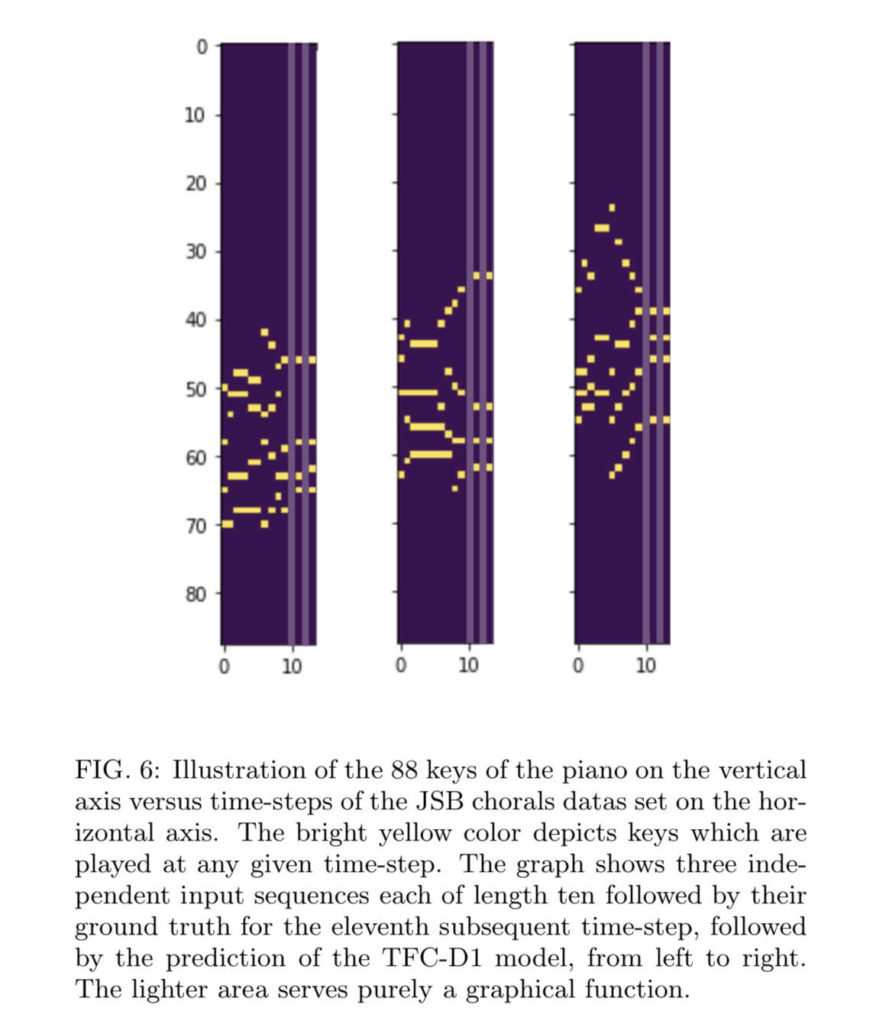
In this work we propose a novel approach to utilize convolutional neural networks for time series forecasting.  We empirically compare this strategy to convolutional LSTM’s and LSTM’s on their performance on the sequential MNIST and the JSB chorals dataset, respectively. We conclude that our  proposed temporally folded convolutional neural networks (TFC’s) may outperform the conventional recurrent strategies.
 

Recent Publication String theory- here

The main focus of my string theory research is to analyze various model independent aspects of quantum gravity effective field theories.

The imprint of the string length modifies the four-four-dimensional theory of our universe and I show that string theory thus might exhibit a rich landscape of de Sitter vacua i.e. describe our universe. Furthermore, this finite imprint of the string length also suffices to describe inflation – the rapid expansion of the universe from a ‘grain of sand’ to about the size it exhibits today. The goal of this work is to advocate the study of alpha’ correction as they can lead to a broader understanding of the string swampland.

Here two results:

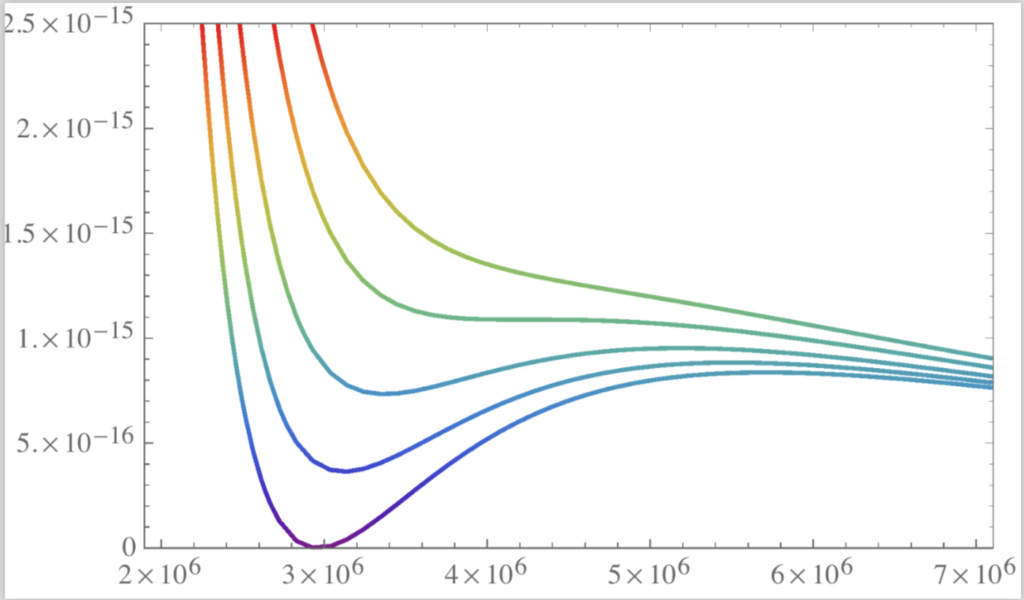
  • The potential derived in the one-modulus case (y-axis the Potential  over the all volume in units of  length  of 2 pi alpha’)
  • And the observable tensor-to-scalar ration r vs. the spectral index n_s (the color coding is the distance in field-space during inflation). The plot is for 60 e-foldings. The colored region can be accessed with the model. For 50 e-foldings the upper bound approaches r < 0.0012.

Selected Recent Talks

  • 6 Feb 2020 – Plenary Talk, String Phenomenology Workshop KEK, Japan
  • 29 May 2018 – Theory seminar at SNU,  Seoul
  • 15 Mar. 2018 – Theory seminar at HKUST IAS,  Hong Kong SAR
  • 8 Dec. 2017 –  Plenary Talk, String Theory Workshop, KIAS,  Seoul
  • 7 Sep. 2017 – Seminar at YITP, Stoney Brook University, USA
  • 5 Sep. 2017 – Theory seminar at Cornell University, Ithaca, USA
  • 31 Jul 2017 – Theory seminar at the Max PIanck Institute for Physics in Munich, Germany
  • 6 Jan 2017 – Plenary talk at workshop, theor. Physics dept. Berkeley @ KIPMU week, Berkeley, USA

String Theory Research

To evolve a complete understanding of the vacuum structure of our universe one needs to study an ultraviolet complete theory of all interactions thus in particular a theory of quantum gravity. The starting point for such a study is widely believed to be string theory.

For the advanced reader,  here are some of the question I’m currently  tackling

  • Search and analysis of Strong Gravitational Lenses  using machine learning.
  • Stringy corrections to the Kähler moduli space metric of 4d, N=1  effective supergravity theories in F-theory , and consequences thereof for moduli stabilization and (meta-) stable vacua.
  • The Moduli space metric of theories with 4 supercharges might not be a product of  – Kähler x Complex Structure – globally. Does the metric contain off-diagonal elements at higher-order in the string length?
  • By integrating out massive KK-states  at one-loop  in Calabi-Yau fourfold reductions one obtains remnants in the 3, N=2  & 4d, N=1 supergravity theories?

My research is devoted to gaining full model independent control of the relevant string length and Kaluza Klein quantum induced corrections to the Wilsonian low energy effective field theories of string theory. The phenomenologically relevant low energy effective theories are four-dimensional supersymmetric theories of gravity and other fields such as scalars, vectors, and fermions. The main focus of those studies concern the Kählerpotential of 4d, N=1 and 3d, N=2 supergravity theories, due to their relevance for phemonemlogy of particlephysics and cosmologywithin string theory.  You may also follow me on HEP-Inspire.  or on Research Gate.

2018-Now

  •  In two single author publications I provide evidence for a novel alpha’-correction to the 4d Kähler coordinates which breaks the no-scale structure. The resulting scalar potential from the leading order alpha’-corrections may  suffice to stabilize all Kähler moduli in de Sitter and Minkowski vacua. by tuning of the flux-superpotential.

2017

  •  We discuss the three-dimensional  Kählerpotential & coordinates, the no-scale structure and the resulting scalar potential  obtained from higher-derivatives terms in eleven-dimensions . We cast light on a new elusive correction to the 3d Kähler-coordinates admitting the interpretation of a KK one-loop correction obtained on the circular reduction from four to three dimensions. view publication
  • Revising higher-derivative terms in ten & eleven dimensions. We use supersymmetry in four and five dimensions to draw conclusions about the structure of the ten and eleven-dimensional theories. In fact, we encounter novel eight-derivative terms in ten as well as eleven dimensions, which have been overseen in the literature but are necessary for consistency of the theories. view publication

2016

  • Revisiting the Euler characteristic correction to the 4d, N=1 Kählerpotential for Calabi-Yau threefolds with an arbitrary number of 4-cycles. I.e. the BBHL correction to the Kählerpotential is recomputed for arbitrary number of Kähler moduli. We explicitly derive the metric background by solving the ten-dimensional modified Einstein equations. The resulting internal six-dimenisonal manifold is no longer Ricci -flat, i.e. is no longer Calabi-Yau. view publication
  • Novel  four-derivative terms to four-dimensional theories or in other words  string theory induced friction terms for inflation are computed. More concretely, those are four-derivative terms of an arbitrary number of Kähler deformations of Calabi-Yau threefold which couple to the four-dimensional Ricci tensor. Those couplings favour slow role inflation when the role of the  inflaton is played by one of the Kähler moduli.   view publication

2015

  • My Ph.D. thesis on geometric corrections to string effective actions. New results include higher-deriviative induced terms of complex structure  deformations of the internal manifold. Those correspond to deformations of 4-cycles of the internal fourfold. Also the imprint of  5-cycle deformations on the effective theory are presented.  view publication
  • The effective action of warped M-theory reductions with higher-derivative terms – Part II .  Following up the previous – Part I – we show that the warp factor in full generality may be integrated into a Kählerpotential. We furthermore, suggest that  the Kähler-coordinates  i.e. the complex structure on the Kähler moduli space needs to be expressed as divisor integrals of the  compactification fourfold. view publication

2014

  • The effective action of warped M-theory reductions with higher-derivative terms – Part I. The effective three-dimensional theory including the dynamical imprint of deformations of an arbitrary number of 6-cycles in the internal space is derived. view publication
  • We reexamine M-theory warped fourfold vacua in the presence of higher-curvature terms. The modified Einstein equations @ eight-derivatives are solved explicitly for the  new background metric. The internal space is no longer Calabi-Yau, thus no longer Ricci-flat. Furthermore, we find that the 5th torsion class is non-vanishing.  view publication

    2013

    • We analyze the hypothetical uplift – in F-theory – of a novel topological correction from a three-dimensional theory to four dimensions. The previously derived correction in three dimension given by the third Chern form of the internal manifold when naively uplifted to four dimensions simply counts the number of the self intersections of present stacks of D7 branes. view publication
    • We derive a novel  correction to the 3d, N=2 Kählerpotential given by the third Chern form of the internal Calabi-Yau fourfold. Uplifted to four dimensions in the framework of F-theory this results in a leading order alpha prime squared – the fourth power in the string length – correction. view publication

Outreach

My recent and most memorable public outreach

 

Co-hosting Communication Skill Session for businessman, entrepreneurs and investors within the Executive Management Program,  University of Tokyo – 2018, November

 

Public Talk at the Tamarokuto Science Center , Event Hall  -2018, July Title: Quantum Strings and the Vacuum Structure of the Universe

 

Munich Science Slam 2013 (winner) – A comedian 10 minute play about string theory (TV-broadcast).

 

Representing the Max-Planck society @ Prince of Asturias award 2013. My outreach in this week contained a TV interview about the importance of  international collaborations in science as well as  a TV broadcasted podium discussion about  the same topic. The highlight of the week was  the award ceremony.

Teaching

  • Co-founder of the “ForscherInnen-werkstatt Physik” in 2007, a program where interested high-school scholars of age 12 to 15 could sign up to participate in experiments held at the university of Graz.  I am happy to announce that the “ForscherInnen-werkstatt Physik” had been successfully continued. The experiments were designed analogously to the “Physics Olympiad” programs which exists worldwide, but with a strong focus on hands on experiments.

Machine Learning & Computational Physics

Machine Learning – Time Series Forecasting

My recent advances lie in the filed of machine learning in time series forecasting, see here .

In this work we propose a novel approach to utilize convolutional neural networks for time series forecasting.  We empirically compare this strategy to convolutional LSTM’s and LSTM’s on their performance on the sequential MNIST and the JSB chorals dataset, respectively. We conclude that our  proposed temporally folded convolutional neural networks (TFC’s) may outperform the conventional recurrent strategies.

The Tensor Calculus Package

The XTensor (xAct) package is an Algebra package for abstract tensor computations in arbitrary dimensions.

The XArus package (currently non-publicly available) was developed independently during the last years, and extends the XTensor package to be applicable on complex manifolds of arbitrary even dimension.

Furthermore, the code allows for non-trivially manipulations of higher-derivative  Lagrangians and E.O.M’s.

One of the most powerful segments is the possibility of generating all Shouten identities and total derivative identities of a given set of higher-derivative operators, as well as Bianchi identities when gravity is involved. This allows to perform non-trivial manipulations of the action. As those analytical identities needed to answer questions relevant in our research can easily grow in the order of thousands we heavily rely on the support of XArus.

Most prominently the package is thus ideal to perform compactifications of higher-derivative supergravity theories relevant in string theory.

Machine Learning – Gravitational Lensing.

Picture deep learning algorithms (AI) are ideal for the search for gravitational lenses, however have been rarely implemented in this context so far. Instead this challenging tasks are mostly performed by conventional algorithms. When a massive object like a galaxy is covering another galaxy in the line of view light bends around the lensing galaxy and becomes thus visible to us. The goal is to try to outsmart conventional algorithms with deep learning image recognition analysis to find many more gravitational lenses in observational images  of our universe within the HSC data of the Subaru telescope which have remained undiscovered so far.

Convolutional Neural Networks have  proven very effective in picture recognition and analysis. To train a custom designed network we need to simulate gravitational lenses.